Progress Report
Actuator Location Optimization for Large Degree-of-Freedom Fields[2] Mathematical Problem Formulation for Actuator Location Optimization
Progress until FY2023
1. Outline of the project
Actuator-position optimization problems are formulated to find actuator positions that efficiently change states such as the temperature and the velocity of the atmosphere. By clarifying the evaluation metrics, it becomes possible to quantitatively evaluate the effect of the actuator placement and to develop an algorithm to determine the actuator placement. If the formulated mathematical problem is too complex, the problem cannot be solved. Hence, we formulate the problems that are simple enough to solve, in coordination with the other themes.
2. Outcome so far
(1)Analysis of actuator-position optimization problems for linear time-invariant systems
Objective functions in the actuator-position optimization problems for linear time-invariant systems were scrutinized by using relationship between objective functions of the actuator-position optimization problems and their dual sensor problems. When any impulse and consecutive-interval inputs is employed and any terminal and consecutive-interval outputs is employed, a matrix representing input-output relationship allows us to evaluate the effect of actuator position. In special cases these matrices coincide with the well-known Gramians in control theory. For example, if we consider a consecutive-interval control input of which norm is limited, the size of the reachable set of states corresponds to the size of the controllability Gramian (Figure 1, top). Furthermore, we investigated metrics to evaluate the magnitude of these matrices and clarified their relationship to existing metrics used in sensor-position optimization and the magnitude relationship between the metrics (Figure 1 bottom).

(2)Formulation of actuator-position optimization problems for nonlinear systems
To account for nonlinearity of weather dynamics, an actuator-position optimization method using the ensemble singular vector method was investigated. This method uses a nonlinear model to make predictions for multiple particles and calculates modes with the greatest input-output sensitivities, allowing for dimensionality reduction. Furthermore, this method has an advantage of not requiring an adjoint model. However, we found that the lack of the use of adjoint models results in a problem: right singular vectors, which are important for determining the actuator position, are not calculated accurately.
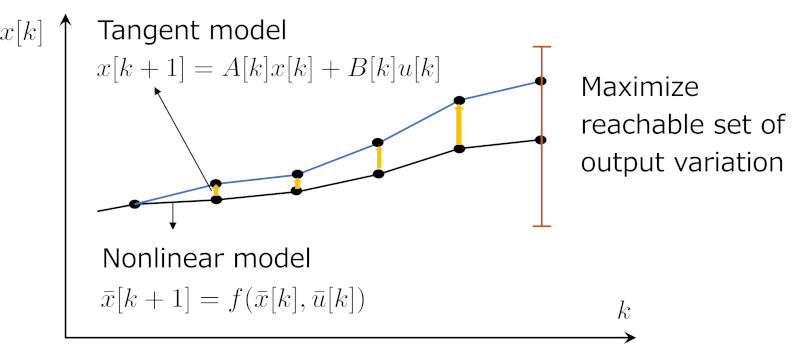
In addition, we formulated an actuator position optimization problem based on tangent linear models. The tangent model describes the variation when a small control input is applied to a nonlinear system. Utilizing the knowledge obtained for linear time-invariant systems in (1), we formulated actuator-position optimization problems in which reachable sets in tangent models, which are linear time-variant, are maximized (Figure 2). Although these problems require the singular value decomposition using adjoint models, it has low computational complexity.
3. Future plans
Given that our project will finish in one year, our project as a whole plans to proceed with an actuator-position optimization problem based on a tangent model in which an adjoint model is used, rather than a problem based on a nonlinear model, which was found to have a challenge in the calculation accuracy of the right singular vectors. On the other hand, since problems based on nonlinear models are important when a weather field changes significantly due to control, we will continue to study problems based on nonlinear models as well as problems based on tangent models in this theme for the future applications.